......

Rubik's Cube is a cube shaped puzzle.  At first glance it consists of 27 cubelets, which make together a 3x3x3-cube.

......

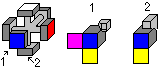In reality there are 21 pieces: 1 main piece with three axles, 8 corner pieces (corner cubelets) with three colours and 12 edge pieces (edge cubelets) with two colours.

The colours of the original cube are white/yellow, orange/red (here: orange=pink) and green/blue. After several random turns the cube is coloured. If the cube is solved, every lateral face has the same colour.  The  main problem is solving the cube.

Solution of the SPIEGEL Magazine slightly altered 
Introductory    top
Ernö Rubik, a Hungarian professor of physics and design, invented the cube in 1974. About 1980 Rubik's cube spread out all over the world like a virus. In the early 80's about 100 million cubes were sold worldwide. People tried to solve it. But most of them kept to one slice or to two slices. 
In Germany the magazine DER SPIEGEL published the first solution, which you could understand. This solution is still of topical interest.
Short after publishing  (4/1981) there appeared a solution for the problem "switching the position of three corner cubelets", which I describe here. You only need 8 moves instead of 22. 
The copyright is at the SPIEGEL and the journalist Albrecht Kunkel.

The following seven pictures show how the cube is solved.
(Picture 4 und picture 6 may be different.)

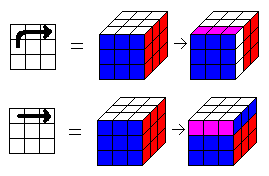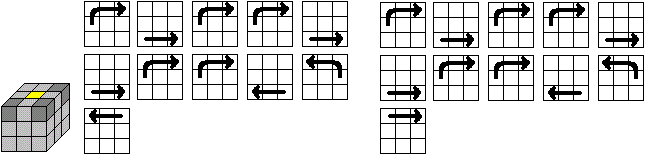
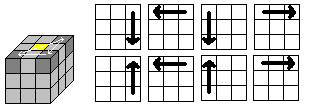
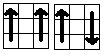
The turns of the slices are shown by a 3x3 square and an arrow. The squares are always the front squares, the arrow describes a quarter turn in direction of the arrowhead. The arrows are in the slice you must turn. The illustration opposite shows the meaning of the squares with arrows in two examples.

 

This is more practical.        .

Remember one principle at the following seven steps of this solution:
Never turn the cube itself but only separate slices during the sequences of turning. That means the orientation of the cube in space is always the same while turning.

Building the First Slice          top
Solving the first and middle slice you have to hold the cube so that the white centre of the face is at the top.

1st step: Solving the edge cubelets.
You look for the edge cubelet blue/white and rotate it to front/down/middle. 
There are two possibilities 1a) or 1b).
1a) white underneath 1b) white in front You repeat the moves with the orange, the green and the red faces in the same way.
Result: On the top there is a white cross. The colours of the edge cubelets and the centre of the four faces have the same colour. (Picture 1 of  7)

2nd step: Solving the corner cubelets.
There are four corner cubelets with the colour white. You hold the cube so that white is at the top and blue in front. 
E.g. you  have to move the corner cubelet white/red/blue to its correct position front/right/top.
First you move this cubelet to the place front/down/left.
There are three possibilities 2a), 2b) or 2c).
2a) white left 2b) white in front 2c) white underneath In the same way you deal with the three remaining white corner cubelets.
Result: The top slice is completely white. The colours of the edge cubelets match the centre cubelets. (Picture 2 of  7)

Building the Middle Slice (3rd step) top
In the middle slice move only four edge cubelets blue/orange, blue/red, green/orange and green/red. You must do this by starting at the bottom. You hold the cube so that white is at the top.
You have to turn the down slice so that one of the edge cubelets blue/orange or blue/red is at front/down/middle. There are two possibilities 3a) or 3b).
3a) to the right 3b) to the left If the blue square shows underneath, you start with another colour.
If all cubelets are in the middle slice, move the cubelet down to the middle by one of the sequences 3a) or 3b) first.
Result: The top slice and the middle slice are in the right positions. (Picture 3 of 7) 

Building the last Slice top
You turn the cube to solve the last slice (white underneath, yellow at the top).

4th step: Switch the position of  two cubelets.
You turn one of the four edge cubelets yellow/blue, yellow/orange, yellow/green, yellow/red to the face with the correct colour (if yellow is not  at the top, don't worry!). If the remaining cubelets are not at the correct positions, you have to switch them over the left edge cubelet at the top. Probably you will have to repeat this sequence.  (Picture 4 of  7) 

5th step: Flip the edge cubelets
Though all edge cubelets are in the correct position,  they might be wrong. Yellow is not at the top.
You have to flip them. You hold the cube so that the edge cubelet is right/top/middle. Now there are eight moves. If the cube is coloured after these 8 turns, go further. Turn the next edge cubelet to be flipped to the same place right/top by turning the whole slice (Don't turn the whole cube). Then there are the eight moves again.
Result: There is a yellow cross. You turn the top slice so that the edge cubelets are at the correct places.  (Picture 4 of  7) 

6th step: Switch the positions of three corner cubelets
First you move the four remaining corner cubelets to the proper positions. Their colours needn't be correct.-
If all four corner cubelets are in the proper position, then the following sequence is unnecessary.
If all the four corner cubelets are at a wrong place after aligning , then there are a further eight moves: If all the four corner cubelets are still in the wrong places, you have to repeat the eight moves. The front face must stay in front.
After these moves one corner cubelet is in a proper place. Turn the cube so that this corner cubelet is back/left. Now you repeat the eight moves. 
Result: The four corner cubelets are in their correct places. (Picture 6 of  7)

7th step: Rotating the corner cubelets.
The colours of a corner cubelet have to turn. 
You hold the cube so that a corner cubelet is top/right/front. Then there are eight moves. If yellow is not at the top of this special corner cubelet, you repeat these eight moves.
Then you turn the top slice (not the whole cube), so that the next corner cubelet is top/right/front. 
You repeat the 8 or 2x8 moves.
If all the four corner cubelets are rotated, yellow must be on the top. Now there is a last step. Turn the last slice. The six faces have one colour. FINISHED!   (Picture 7 of  7) 

Planned Chaos top
Is it possible to turn a cube so that you have every colour on each face at least once? Yes it is.
(Start with blue at the front, white at the top) 
 

Taking to Pieces     top
You can also solve the cube by taking it apart and putting it together.
There are some products with a screw at the centre of a face. You can unscrew it. Then you can solve the cube.
You can take apart most products only with a little force. You turn the top slice about an angle of 45 degrees. You lift the top slice with a screw-driver or a spoon-handle carefully. In this position you can remove an edge cubelet and then the adjacent corner cubelets.

If you are very clever you solve the cube by removing the adhesive films and sticking them again ;-).

Solving with a Stop-Watch    top
Those who can solve the cube see the next challenge: Solving the cube as quickly as possible.
The times solving the cube are different. It depends on the arrangement of the cubelets at the beginning. But there are average times.
The professional cube turners need no more then 90 moves. They know a lot of sequences to enable react them to special situations appropriately. They don't solve the cube step by step. They see many cubelets together.
Besides they use silicone oil.

In the "Guiness Buch der Rekorde 1986" you find:
On June 5, 1982  Min Thai, USA, won the Rubik`s Cube Championship in Budapest. His  time: 22.95 s.

Solving the Cube with Little Numbers of Sequences    top
Most of the people prefer a method with a small number of sequences (not moves). If you solve the cube you are not willing to look at a paper, you solve it by heart.
In this respect the SPIEGEL solution is a good one.
If you have some practice you can order the first slice easily. Step 2c) is not necessary, you can use 2a) and 2b). You need only sequences of no more than eight moves.
Nevertheless you need 120 to 180 moves, because you have to repeat sequences again and again. If you have bad luck you need 75 moves for the last step "Rotating the corner cubelet". The cube can be solved with the Spiegel method in 2 to 3 minutes.

Some Mathematics top
In the book of Trajber (3) there is a mathematical theory of the cube.
You number the squares, which you can move, from 1 to 48 (48 = 6x9-6). A move or a sequence of moves brings you to a new arrangement of the numbers 1 to 48. This is called a "permutation". All the permutations form a "group". 
So instead of looking into Rubik's Cube you can occupy yourself with the group.
One interesting point in the theory of groups is transferred to the cube:
If you repeat a move or a sequence of moves, you always come back to the beginning. 

If you would like to have some exercise with the sequences of the SPIEGEL solution, you do it like this:

......

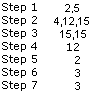You take the solved cube and follow one sequence of moves again and again. After n steps you come back to the clear cube. n is at the second column.

Theoretically there are 54!/(9!*9!*9!*9!*9!*9!) = 1.10^38 combinations of 54 squares of a 3x3x3 cube. 
Rubik's cube shows "only" 8!*3^8*12!*2^12 = 519,024,039,293,878,272,000 =5.19^20 combinations of the 54 squares, if you take apart the cube and put it together. 
43,252,003,274,489,856,000 combinations (1/12) appear at the cube while turning.

Patterns      top
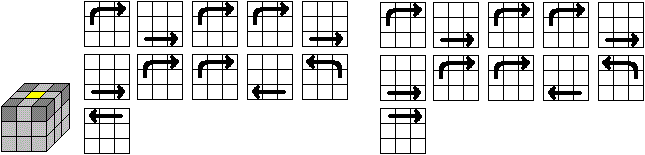
A wide field of activities is finding new patterns.
Here is an example, which you can develop to find more patterns.  (Each arrow means a quarter turn.)

If you repeat the sequence, you return to the solved cube.

Variations:

......

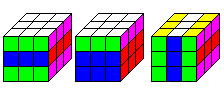If you don't start with the solved cube, but with one variant at the left, you get new patterns.

If you turn the slices in another way, you get new patterns, too.

You get 12 patterns:

Rubik's Cube on the Internet     top

German:

DH-Online-NET
Lösungsweg zur Ordnung des Magischen Würfels (Quelle: Practic-das Magazin der Selbstbautechnik Heft 3/1981)

Jürgen Fischbach
Lösungsseite für den "Rubik's Cube" oder Zauberwürfel (3x3x3)

Lars Petrus
Rubiks Zauberwürfel auf Zeit lösen

Michael Jasmund
Zauberwürfel (Lösung, Spiel, Modelle)

Oliver Reimann 
Anleitung für den Zauberwürfel

Puzzle Shop
Anleitung für den Rubik´s Cube

Ronald Bieber
Rubiks Cube

Sigrun Deweß
Rubik-Zauberwürfel ordnen

Spiegel-Wissen
Schrei Hurra! Schmeiß 'ne Runde!
Die Spiegelausgabe 4/1981 vom 19.01.1981 ist jetzt (Feb2008) für den privaten Gebrauch freigegeben!     :-)

spotn.de (Videos)
Rubiks Cube: Zauberwürfel lösen (Teil 1 von 3), Teil 2, Teil 3

Thomas Stadler
Speedcubing Schweiz

Urs Manser 
Rubik's Cube (Lösung)

Volkmar Grandy
Rubik Jazz

Wikipedia
Zauberwürfel

English:

Georges HELM 
Collection of Rubik's cubes and related puzzles 

Jaap Scherphuis
Rubik's Cube 3x3x3 (Several solutions, Links to other useful pages)

Jessica Fridrich
My speed cubing page

Lars Petrus
Solving Rubik's Cube for speed

Michael Reid   (Englisch)
Rubik's cube information

Nader (naderc)
CV Rubik - Computer sees Rubik's cube and solves it (The Spiegel solution as a video)

Rubik on-line
Ernö Rubik's Official Homepage. Interesting: Cube History

Stefan Pochmann
Stefan Pochmann's Cube Corner

Volkmar Grandy
Rubik Jazz

Wikipedia
Rubik's Cube

References (German)  top
(1) DER SPIEGEL Nr.4/1981
(2) Josef Trajber: Der Würfel, Niedernhausen/Ts. 1981 (Falkenverlag 0565)
(3) Josef Trajber: Der Würfel für Fortgeschrittene, Niedernhausen/Ts. 1981 (Falkenverlag 0590)
(4) Tom Werneck: Der Zauberwürfel, München 1982 (Heyne 4831)
(5) Tom Werneck: Der Zauberwürfel für Könner, München 1982 (Heyne 4834)
(6) Kurt Endl: Rubik's Rätsel des Jahrhunderts, Giessen 1981
(7) Alexander H.Frey, Jr and David Singmaster: Handbook of Cubik Math, Hillside New Jersey1982 [ISBN 0-89490-060-9]